🔎 Understanding WACC

❔ We learned how to calculate WACC in class, but I thought that we did not hear much about what it means and where we use it. For example, in the last slide of PPT, we saw a variety of corporations’ WACC. What information can we get from them? Is the WACC lower the better or higher the better? if so, why? etc.

See answer

✔ Throughout the course, we’ve taken a discount rate for granted.

WACC is how you calculate the discount rate required by your investors for your firm.

You use the WACC when you calculate NPV of projects that you are considering taking on.

WACC is the ‘missing factor’ that is needed for an NPV analysis.

What is the WACC

We have seen that firms use NPV to decide which projects to invest in. How do they determine the discount factor to use in their $NPV$ calculations. The discount rate that we use is the Weighted Average Cost of Capital.

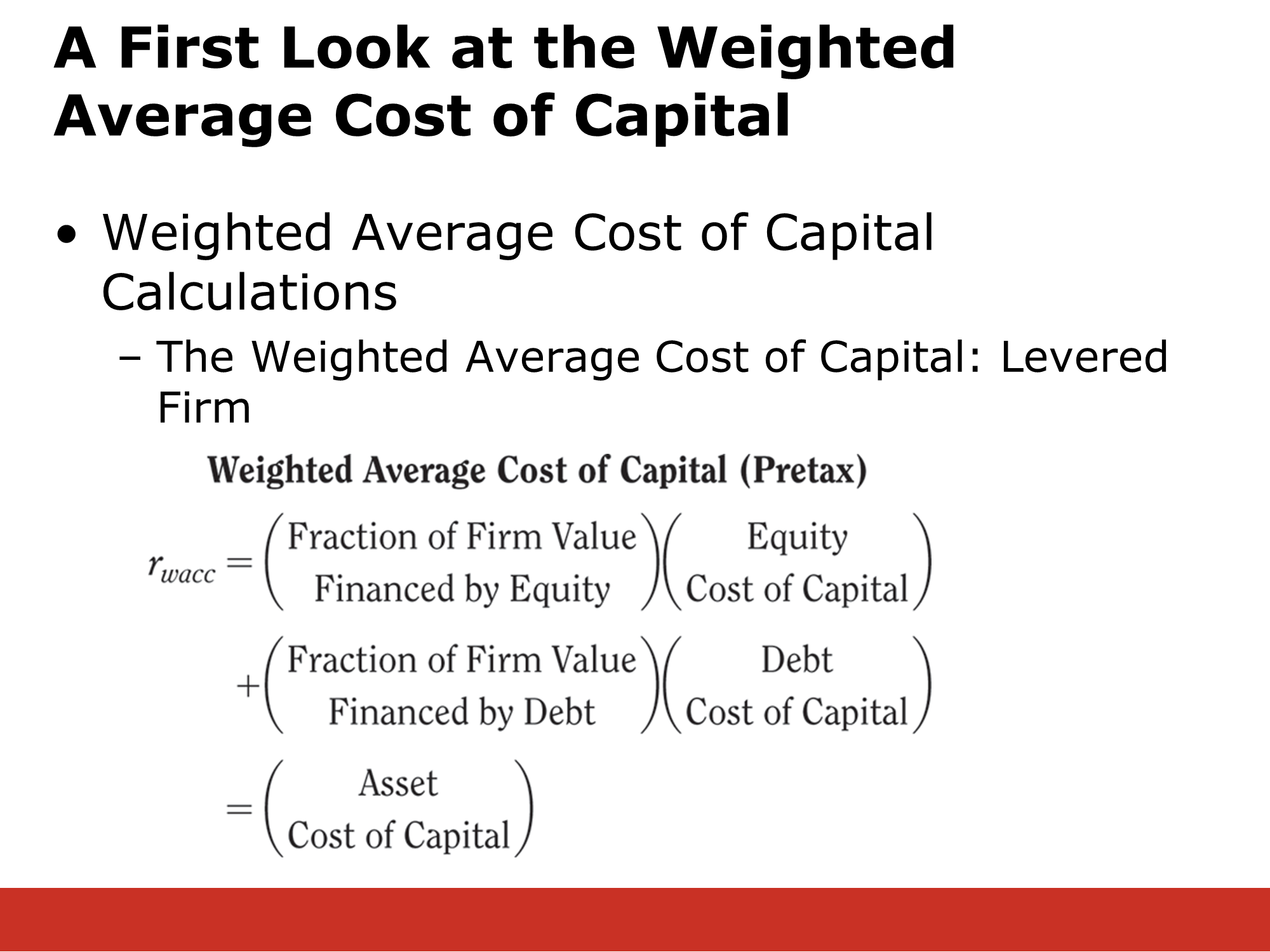
Or, more simply, using $w$ for the weighting factors: $r_{wacc}=w_e r_e+w_d r_d (1−T_C)+w_p r_p$

If your firm is financed solely by equity (unlevered), then your $r_{wacc} = r_e$

If your financing structure is more complicated, you must include the costs of capital for debt and preferred as well.

When you prepare for the final, your first concern is being able to use this formula.

The weighted average that we use in grading is similar: (PS=“Problem Set”, MT=“Midterm”, FE=“Final Exam”) $SemesterScore = w_{PS} avg_{PS} + w_{MT} avg_{MT} + w_{FE} avg_{FE}$
$w_{PS} = 30\%$
$w_{MT} = 30\% \;or \;0\%$
$w_{FE} = 40\% \;or \;70\%$

See an example →

✏️ To calculate your grade, we take a weighted average of your problem set, midterm, and final exam scores:

Suppose your PS Score is $93\%$, your midterm score is $90\%$, and your final is $87\%$.

Suppose your scores are as follows:

	Scores
Problem Set	$93\%$
Midterm	$90\%$
Final	$87\%$

What is your weighted average, using the weights above?

✔ Click here to view answer

For each of the scores, we multiply those scores times the weight. The individual weights must be between $0\%$ and $100\%$, and if you add all of the weights together, you get $100\%$.

$$\begin{aligned} Weighted \;Average &:= PSWeight \times PS \;Score + MidtermWeight \times MidtermScore + FinalWeight \times FinalScore \\ &= 30\% \times 93\% + 30\% \times 90\% + 40\% \times 87\% = 89.7\% \end{aligned}$$

For the WACC the weights are just “The Fraction of Firm Value” financed by a given source of financing.

🧠 Intuition: Weighted Average
Suppose $10\%$ of your funding comes from debt and $90\%$ of your funding is equity. Suppose also that your debt cost of capital is $5\%$ (after taxes) and your equity cost of capital is $15\%$.

✏️ Should your “Weighted Average Cost of Capital” ($WACC$) be closer to $5\%$ or closer to $15\%$? Why? Let’s draw out the intuition of what a “Weighted Average” is.

✔ Click here to view answer

Your WACC will be closer to the cost of capital of equity because your firm is $90\%$ funded by equity.
Because you only added $10\%$ debt funding to an organization funded otherwise by equity, you can think of it as follows: visualizing a numberline, start at the Equity CC ($15\%$) and go $10\%$ of the distance to the Debt CC ($5\%$). The distance between the Equity CC and Debt CC is 10 percentage points. $10\%$ of that is one percentage point. Therefore, you start at the Equity CC ($15\%$) and decrease that number by 1 percentage point to get $14\%$ as the weighted average, without even using the formula!

Now that we’ve done the important stuff (the intuition), let’s do the calculation. The calculation will get you most of the points on the exam, but leaders should understand the intuition as well. (It also helps you remember things and work quickly through exam questions, because everything makes more sense.)

✏️ What is the WACC?

✔ Click here to view answer

$WACC = 90\% \times 15\% + 10\% \times 5\% = 14.0\%$
Note that $14\%$ is $10\%$ of the way from $15\%$ to $5\%$.

The remainder of this lecture is about calculating the $w_e$, $w_d$, $w_p$, and the $r_e$, $r_d$, $r_p$…

How do you calculate the weights? ($w_e$, $w_d$, $w_p$)

The weights are the percentages of your financing that comes from $e$, $d$, or $p$.

Consider a firm with the following market value balance sheet:

Assets	Liabilities + Stockholder Equity
	$Debt \;MV_d = \$3M$ (No liabilities other than debt.) $Equity: MV_e = \$2M$ $Preferred \;Equity: MV_p = \$1M$

🧠 Important Intuition: this is very helpful for understanding the equations for these weights and also the key results from two of the following lectures: M&M theorem and Mergers & Acquisitions. It’s an important part of what I refer to as “The Corporate Finance Perspective.”

✏️ If investors are willing to pay $\$3M$, $\$2M$, and $\$1M$ for the firm’s debt, equity, and preferred equity, how much do you think they would pay for the firm’s assets as a whole? In other words, how much is the entire firm worth?

✔ Click here to view answer

Recall the fundamental accounting equation:
 $Assets = Liabilities + Stockholder \;Equity$
The market value of the firm’s assets, then, will be
 $MV_A = MV_L + MV_{SHE}$
Using the data from our table, we get:
 $MV_A = \$3M + (\$2M+\$1M) = \$6M = the \;market \;value \;of \;all \;financing$
 $MV = "Market \;Value"$
We foreshadowed this idea when covering “What is Enterprise Value?”

The value of the Assets of a firm equals the value of all claims (liabilities & equity) on those assets.

$Total \;financing = \$1M + \$2M + \$3M = \$6M = total \;value \;of assets.$

$w_p= \frac{1M}{6M} = \frac{1}{6} =0.1667$

$w_e= \frac{2M}{6M} = \frac{2}{6} = 0.3333$

$w_d= \frac{3M}{6M} = \frac{3}{6} = 0.5$

We always prefer using market value, but, occasionally, since debt markets can be opaque, we might be stuck using book value for debt.

How do you calculate $r_p$?

For the cost of capital for preferred equity, we just calculate the return on preferred equity.

$r_p = \frac{dividend \;payment}{price}$

Suppose I have preferred shares with a market value of $\$26$ and they pay a $\$2.20$ divided every year.

$r_p = \frac{2.2}{26} = 0.0846$

How do you calculate $r_d$?

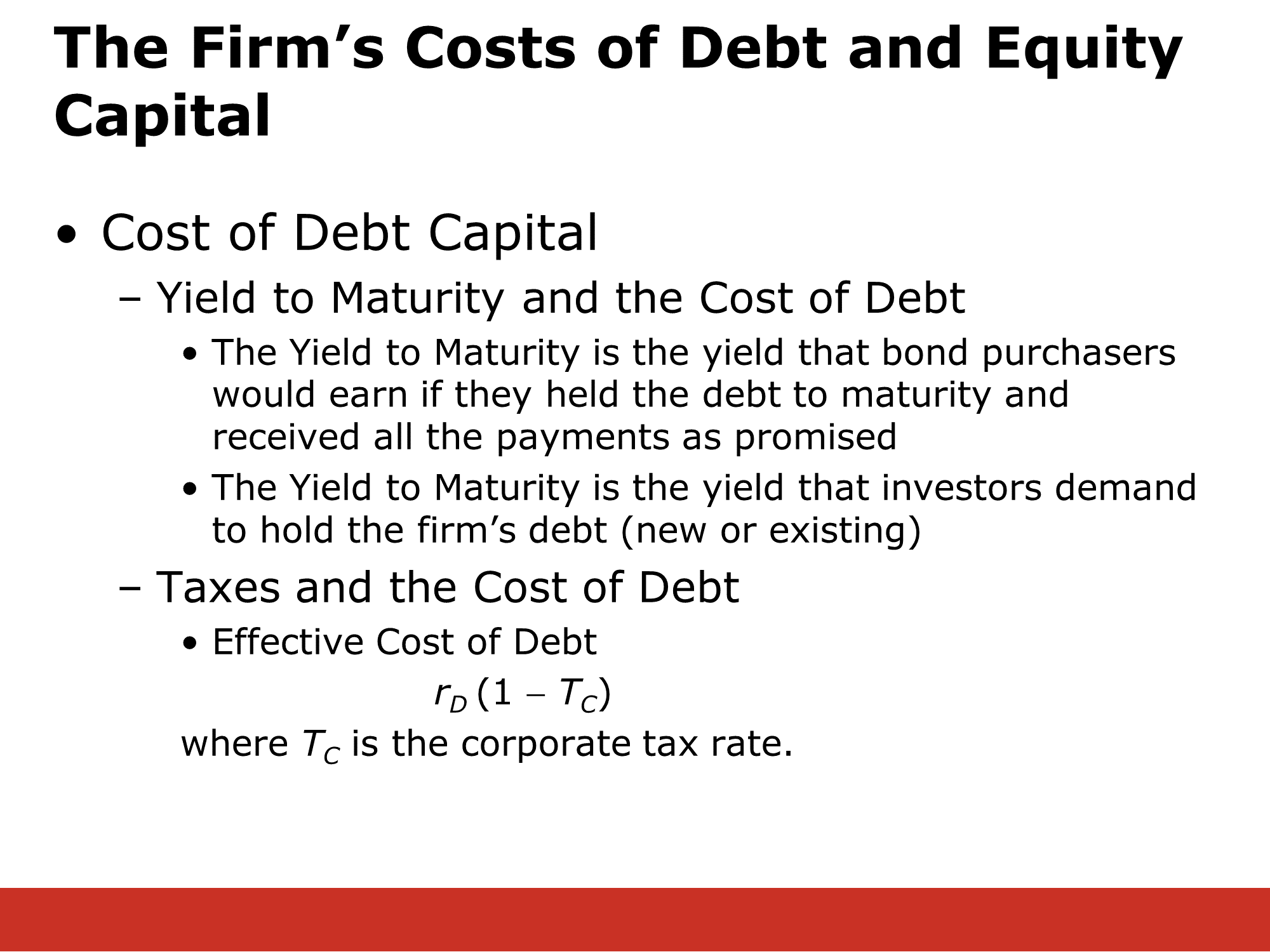
To calculate out $r_d$, the cost of debt, typically, you’ll be given the $YTM$ or may have to calculate the $YTM$ using the techniques from the lecture on Debt Issuance. One key trick that can be helpful is that when any security is selling at face value (aka ‘par value’), its $YTM$ is just its coupon rate.

$Effective \;cost \;of \;debt = r_d(1-T_C)$

The pretax cost of capital, $r_d$, is just the $YTM$. However, if you have a marginal tax rate of $35\%$, then when you pay $\$1$ of interest, you get a $\$0.35$ decrease in your taxes. Thus, the effective cost of debt is only $\$0.65$, or $65\% = (1-T_C)$ of the $YTM$.

Bottom line: $Effective \;cost \;of \;debt \;capital = r_d(1-T_C)$, where $r_d$ is the pretax $YTM$.

❔ If they are now issuing new debt at a rate but issued old debt at an unknown rate, how do we treat that unknown rate?

See answer

✔ Generally, there will only be one $r_d$, so can use either debt issuance to find $r_d$. Usually, you can only calculate the yield of one bond, so use that as the $r_d$.

How do you calculate $r_e$?

There are two ways: using the CAPM and using Constant Dividend Growth Model.

CAPM

Your formula is: $equity \;cost \;of \;capital = r_e = r_F+β[E(r_M)−r_F]$

$β$ is the risk that your stock adds to a well-diversified portfolio.

$E(r_M)−r_F$ is the (Expected) Excess Return of the market (portfolio) or Market Risk Premium.
Jargon hint: In general, the words “Excess” or “Premium” means that you are subtracting off $r_F$, because you are looking for the premium above $r_F$. $E(r_M)$ is the Expected Return of the market. Because we don’t know what the market return will actually be, we use the “Expected Return.” Expected return is defined mathematically, and you will just be given it.

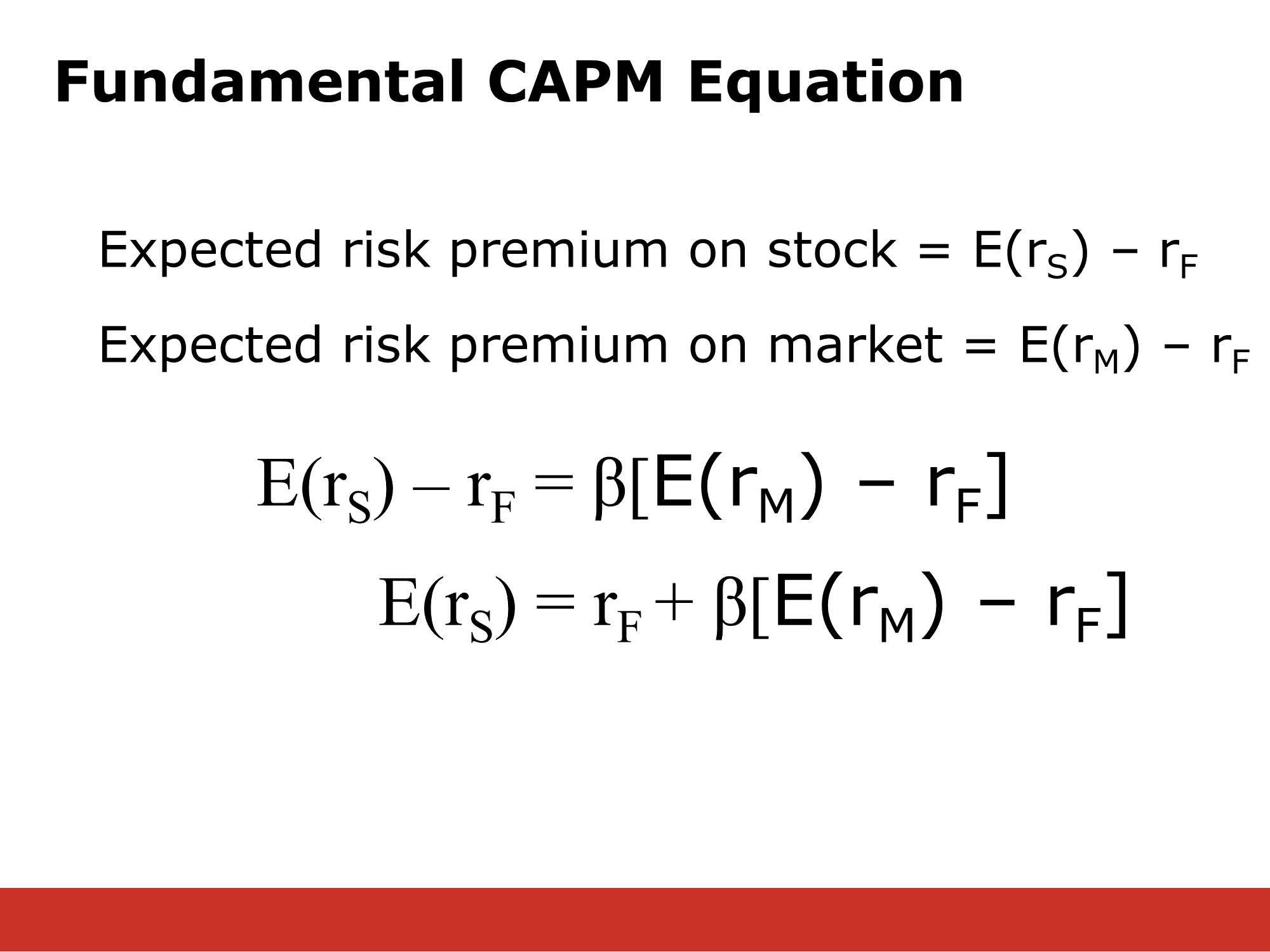
Constant Dividend Growth Model

$$r_e = DivYield+DivGrowth=\frac{Div}{P}+DivGrowthRate$$